Pure Moments and Couples

What happens if you have two equal and opposite forces acting on an object along the same line of action? Nothing, right? The forces sum up to zero.

Ok, now imagine that these forces aren't acting on the same line of action. Imagine that someone is pushing on both parts of the above triangle. Naturally, you know that the triangle is going to rotate in the clockwise direction. This is called a force couple.

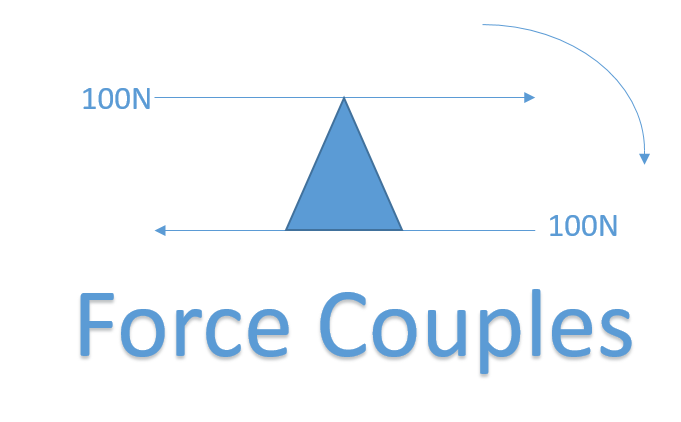
The moment produced by two equal, opposite, and non-collinear forces is called a couple.

Let's break that definition apart. As we discussed in a previous article, a moment is the tendency of a force to rotate an object around an axis. For a point to be collinear, it has to lie along the same line as the reference point.

Another key idea behind a force couple is that it doesn't cause translation to occur. That means the total sum of forces has to balance out to zero so that the object doesn't move.

The best example I can come up with for a force moment is the rotation of a screwdriver. It has a force applied from each finger that perfectly balances out so that you aren't driving the screw in unevenly.

Since this is an engineering blog, we have to do a little math.

Force moment = r x F
where r is the force vector, x symbolizes the cross production operation, and F is the force.

This can be simplified to M=Fd where the moment is equal to force multiplied by distance.

Let's try a simple couple problem.

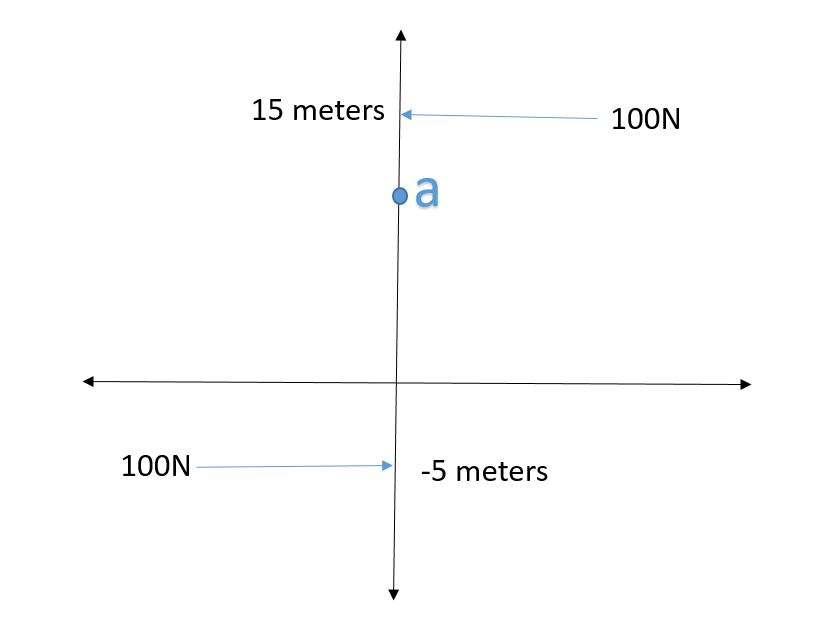
Here we see that point "a" has two opposing and non-collinear forces acting upon it.

These forces can be rearranged as a single torque.

How?

We take the total displacement of the forces and calculate the torque using just one of the force vectors.

This will give us a torque value for our couple.

The two forces are 20 meters apart.

This gives us:

M = Fd = 100N*20m = 2,000N-m

By inspection, we see that the direction of rotation is counter-clockwise.

For the sake of completeness, force couples can be combined with other forces to form an overall resultant force on the system.

If we included a 100N force applied in the Y direction, we'd have a net 100N of translational force and 200Nm CCW of rotational force

As always, be sure to check out our other articles on Introduction to Statics, Static Forces, and Rotational Force.